Introduction to Antilog: Properties, Applications, & Examples 

In Mathematics, Antilog is a technique through which we can find the inverse power of any number without any problem. This terminology plays an important role in calculating the least and the most values while calculations. 

Sometimes, you have to find the 1/nth root of a specific number. If you made simple calculations to find it, then this will be time taking and maybe boring. In this topic, we will learn a new concept of Anti-Logarithms (antilog). Antilog has vast applications in the field of Math.

What is Antilog?

Antilog sometimes pronounces as ‘‘Anti-Logarithms ’’ of a number, is the reverse process to find a logarithm of the given number. Both logarithm and Anti-Logarithm are related to each other. Let’s suppose x is the logarithm of the number y with b as a base, then we can say that y is the antilog of x to b. It is written as 

If,

log y = x 

Then,

y = antilog x

How to find the antilogarithm of a number?

While finding the antilog of a number, one’s should be aware of the characteristic and mantissa part of a number. These two parts are explained below:

Characteristic part: The part that comes before the decimal point is called Characteristic of a number. In other words, we can define the Characteristic such as the whole number part of any number is known as the characteristic part. Make sure that the characteristic of the logarithm of a number that is bigger than one is positive and is one unit lesser than the total number of digits there on the left side of the decimal point.

Mantissa part: It is defined as the decimal part of the logarithm of a number known as Mantissa. It should be bigger than 0, i.e., it must be a positive number. When a mantissa part is a negative number or less than zero, we convert it into a positive one.

Steps:

The Logarithms and Anti-Logarithms with 10 as a base can be changed into natural Logarithms. As we know that both Natural Logarithms and Anti-Logarithms have their base as 2.7183. Similarly, Anti-Logarithms may be changed to Natural Logarithms by multiplying it by 2.303.

While finding the anti-logarithm of a number we use an anti-logarithmic table. Here are some steps to find out the antilog.

• The first step is to sort out the characteristic and the mantissa part of the given number.
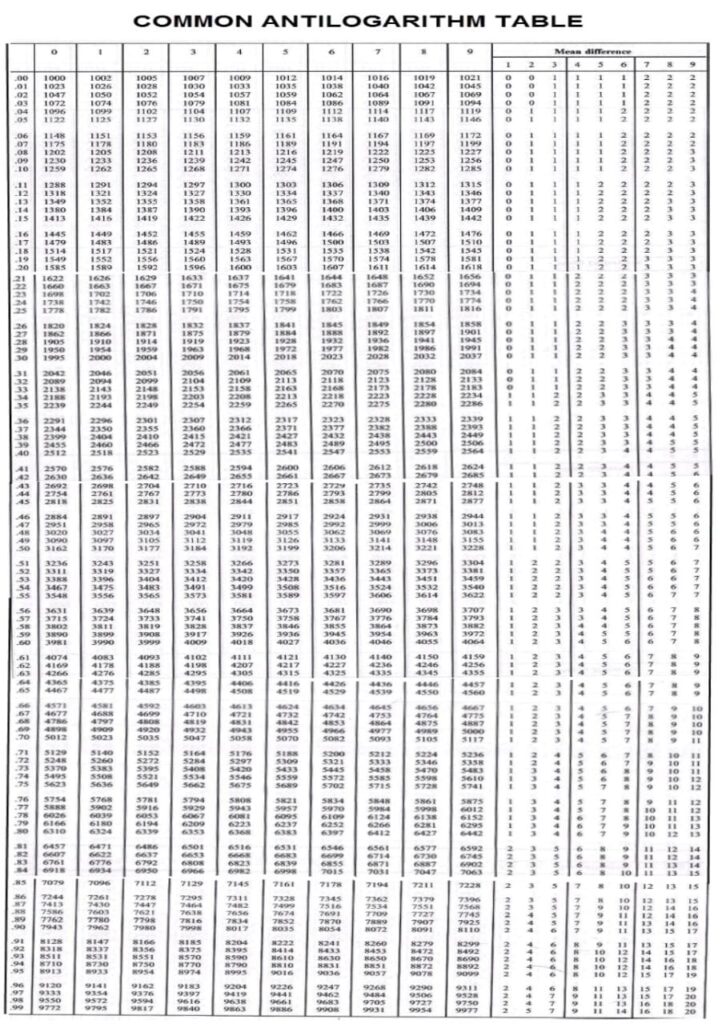
• The second step is to use the antilog table to find a corresponding value for the mantissa. The first two digits of the mantissa functioned as the row number and the third digit is equal to the column number. Note the value of it.
• The table of antilog may also be including columns that impart the mean difference. Here for the same row of the mantissa, the column number in the mean difference is exactly equal to the digit in the fourth place. Write this value.
• Add the values to obtain.
• In the characteristic, you have to add one. This value represents the place to put the decimal point. The decimal point is to put after that many digits from the left.

How to use an antilog table?

An Antilogarithm table usually calculates the logarithm of some numbers. As provided the logarithm of any number can be studied (characteristic and mantissa), where the characteristic may be positive or negative whereas the mantissa should constantly be positive.

 Consider we know how to separate the characteristic and mantissa from the logarithm of a number. Usually, we have to be careful while solving through this method of negative numbers.

A Swiss mathematician Jost Bürgi independently of John Napier, who constructed the logarithmic table in 1614, first introduced the concept of the table of antilog.

Examples:

Here are a few examples to learn how to calculate antilog problems.

Example 1: 

Calculate the antilog of 4.8551.

Solution:

Here first, we have to find the number whose logarithm is 4.855.

From the antilog table, the value corresponding to row 85 and column 5 is 7161. 

The mean difference column for the value 1 is 2.

Adding these two values, we have 7161 + 2 = 7163.

The decimal point is placed in 4 + 1 = 5 digits from the left. 

So, antilog 4.8551= 71630.0

Example 2: 

Calculate the antilog of 1.2344. 

Solution:

First, we need to find the number whose logarithm is 1.2344.

From the antilog table, the value corresponding to row 23 and column 4 is 1714.

The mean difference column for the value 4 is 2. 

Adding these two values, we have 1714 + 2 = 1716.

The decimal point is placed in 1 + 1 = 4 digits from the left.

 So, antilog 1.2344= 17.16

Example 3: 

Suppose a colony of bacteria is growing rapidly. Currently, the colony is 6 times as large as it was 5 days ago. How much time is required for doubling? 

Solution:

 Remember   the doubling formula that is given by:

 A(t) = Ao · 2t/k 

Here we should have to know the fact that after 5 days the colony is 6 times as large implies that

 When

t = 5, A(5) = 6Ao

 So,

 6 Ao = Ao · 25/k 

We then want to solve for k:

 6Ao = Ao · 25/k

 ⇒ 6 = 25/k

 ⇒ log(6)  = log(25/k) 

⇒ log(6) = 5/k [ log(2)]

 ⇒ k = 5log(2)/ log(6)

⇒ k = 5 log(2)/log(2)log(3)

⇒ k= 5/log(3)

⇒ k= 5/0.4771

⇒ k= 10.48

Example 4: 

A specific radioactive isotope decays exponentially with a half-life of 68 years. If we start with 21 grams of the isotope, how many years will it take until there are 7 grams left? 

 Solution: 

Using our half-life equation, we get that

A(t) = 21 × [½]t/68 

Here we have to solve for the t such that

A(t) = 7

 Putting 7 for A(t) is the required equation and solving gives:

 7 = 21 × [½]t/68

 ⇒  7/21 = [½]t/68

⇒ log( 1/3 ) = log[½]t/68

 ⇒ log(1/3 ) = [t/68]log[½]

⇒ t = [(68) log( 1/3 )] / log[½]

 Applications

In Physics: Many physical phenomenon are those in which the role of logarithm and antilog is too much crucial like the magnitude of the earthquake. In this phenomenon, the technique of logarithm and antilog is used for measuring the magnitude of earthquakes on the Richter scale. 

Moreover, to calculate the sound intensity level, we have to take into mind the concept of logarithm and antilog. The formula for sound intensity level is given as fellow:

Sound intensity level=10 log I/IO

    L – LO=   10 log I/IO

In Chemistry: In many chemical reactions, the concept of logarithms and antilog is used in finding the pH (to check whether the chemical is acidic or basic). 

pH = log10 [H+]

Similarly, many types of catalysts are used to proceed with the chemical reaction as early as possible. The quantity of catalyst tells us about the speed of the reaction. For using the least or the most quantity of catalyst, the concept of antilog and logarithm must be taken into mind.

In Finance:  Many well-known Logarithmic price scales are used in plotting charts in such a way that two equivalent price changes are shown by the same vertical changes on the scale. By using this we acknowledge the analysis of price changes.

In Engineering: In this field, the concept of antilog is widely used such as technological engineering. In this, engineers use many precisions in numbers after many time intervals to check the reliability of a specific tool or machine.

In Biology: Biomedical engineers use the techniques and concept of antilog to find out the period of cell decay and growth, and also to measure the effect of light intensity on bones and to avoid UV radiations.

In number precisions: It is widely used when numbers are too large or too small to handle easily. Like in astronomy, many calculations are too large or too small, without using the concept of logarithm and antilog, it is difficult to find a timely solution. 

Conclusion:

In this topic, we recognize the concept of antilog and its relation with logarithm by knowing its introduction, table, examples, world problems, and daily life application. Through its concept, we can easily handle all kinds of problems related to it.

Disclaimer: quizsansar.com does not own this book, PDF Materials Images, neither created nor scanned. The material and information contained on this website is for general information purposes only. If you have a complaint about something or find your content is being used incorrectly then kindly mail us: sansarquiz@gmail.com

Arithmetic Sequence: An Introduction with Calculations

Arithmetic Sequence: An Introduction with Calculations

A sequence is a group of numbers, events, or movements that follows a specific pattern. Class roll number and the days in the year are the best examples of sequence. The sequence has two types; finite and infinite sequence.

The finite sequence consists of the last term. For example set of the first ten even numbers. A sequence that has no end term is known as an infinite sequence. For example set of Natural numbers.

A sequence in which the difference between two consecutive numbers remains fixed is known as an arithmetic sequence. For example set of Even numbers. In this article, we will discuss the definition of Arithmetic sequence and its formulas. At last, we will discuss some examples related to the Arithmetic sequence.

Definition of Arithmetic sequence

A sequence is an arithmetic sequence if the difference between every consecutive term remains the same. In other words, in the arithmetic sequence, we add or subtract any number in sequence to get the next terms. An arithmetic sequence is also known as Arithmetic progression (A.P), For example

4, 9, 14, 19, 24…

You can observe that difference between every two consecutive pairs is 5. Or by adding 4, we obtain the next term.

Usually, Sequences are denoted by English letters a, b, c, etc. In a sequence, we use n for the variable instead of x. The standard form of the sequence is a₁, a₂, a₃… a_n. where a₁ is the first term and a_n is called the last term of the sequence.

Formulas for the Arithmetic sequence to calculate the n^th term

We have two formulas to find the n^th term of the arithmetic sequence we use the following formula

• a_n = a₁ + (n – 1) d for all n > 1
• a_n = d + a_n-1

a_n = a₁ + (n – 1) d for all n > 1 is the general form of A.P, where a₁ is the first term and a_n is the n^th term that we want to calculate, n is the total term in the Arithmetic sequence and d is the common difference between two consecutive numbers i.e. d = a_n – a_n-1  for all n > 0. It is useful when the first term of the sequence is given.

a_n = d + a_n-1 is the recursive formula for an arithmetic sequence. It is useful when the first term a₁ is not given. For example, find the a₂₀ term when a₁₉ = 3   and the common difference is 2. Then simply we put the values and calculate the n^th term.

Formula to calculate the sum of n term

We have 2 following formulas to find the sum of the n term of the arithmetic sequence depending on the condition.

• S_n= n/2[2a₁ + (n − 1) × d] for all n > 0
• S_n= (n / 2) (a₁ + a_n)

if we have only 1^st term of the arithmetic sequence but do not have the last term then we use

S_n = n/2[2a₁ + (n − 1) × d] for all n > 0

But if the last term and 1^st term are given then we use S_n = (n / 2) (a₁ + a_n)

For the calculations of nth term and the sum of a sequence, an arithmetic sequence calculator can be used as it will given the solution with steps in a fraction of a second.

Solved examples

Example 1:

Find the general term and 9^th term of A.P. if the first term is 2 and the common difference is 5.

Solution:

Step 1: Identify the given data,

Here a₁ = 2 and d = 5

Step 2: Write the formula of A.P i.e.

a_n = a₁ + (n – 1) d

Step 3: Now put the given data into the formula,

a_n = 2 + (n – 1) 5

Step 4: Simplify

a_n = 2 + 5n – 5

a_n = 5n – 3 (That is the required general form of A.P)

Step 5: Since we need the 9^th term so we take n = 9 and put in a_n = 5n – 3

a₉ = 5(9) – 3

a₉ = 45 – 3 = 42

Example 2:

Find the sum of the first 50 natural numbers.

Solution:

First 50 natural numbers = {1, 2, 3, 4, 5… 50}. Since we have both first and last terms so we use the following formula to find the sum of the first 50 natural number

S_n = (n / 2) (a₁ + a_n)

In this example total terms = n =50, the first term = a₁ = 1, and the last term = a_n = 50. Now put these values in the above formula

S₅₀ = (50 / 2) (1 + 50)

S₅₀ = (50 / 2) (51)

S₅₀ = (25) (51) = 1275

So the sum of the first 50 natural numbers is equal to 1275.

Example 3:

Find the number of the term in the Arithmetic sequence. If a₁ = 4 and d = 2 and a_n = 40

Solution:

By using a_n = a₁ + (n – 1) d, we have

40 = 4 + (n – 1)2

40 = 4 + 2n – 2

40 = 2 + 2n

40 – 2 = 2n

38 = 2n

n = 38 / 2 = 19

Conclusion

In this article, first, we discussed the definition of sequence and its types with examples. Then we explained the arithmetic sequence with its example. The formulas to calculate the n^th term and sum of the arithmetic sequence and important notations are also covered in this article. We learned when these formulas would be applied.

Disclaimer: quizsansar.com does not own this book, PDF Materials Images, neither created nor scanned. The material and information contained on this website is for general information purposes only. If you have a complaint about something or find your content is being used incorrectly then kindly mail us: sansarquiz@gmail.com